Explicit Differentiation
Explicit function: If ![]() is easily expressed in term of the independent variables
is easily expressed in term of the independent variables ![]() ,Then
,Then ![]() is called an Explicit function of
is called an Explicit function of ![]() . Symbolically it is written as
. Symbolically it is written as ![]()
Examples:
![]()
![]()
![]()
Procedure:
- Step (1) when
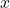 and
and 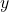 are not amalgamated or Explicit we assumethat
are not amalgamated or Explicit we assumethat 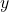 is differentiable function of
is differentiable function of 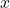 .
. - Step (2) Differentiate both sides of eq w.r.t
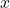 .
. - Step (3) Solve the resulting eq for
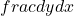

Example 1:
Explicit Differentiation
![]()
Differentiate w.r.t ![]()
![]()
![]()
![]()
![]()
![]()
Example 2:
Explicit Differentiation.

![]()
Differentiate w.r.t ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Example 3:

Explicit Differentiation
![]()
Differentiate w.r.t ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
