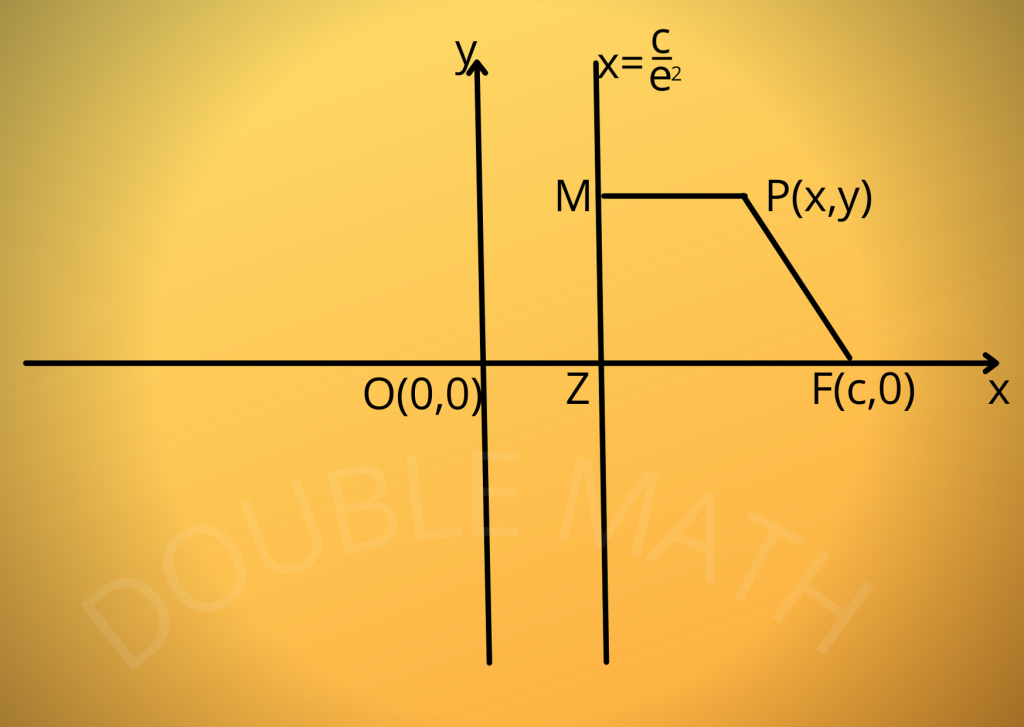
What is Hyperbola, Graph, and Equation

What is hyperbola:We have already stated that a conic section is a hyperbola if $e > 1$ you can check. Let $e > 1$ and $F$ be a fixed point and $L$ be a line not containing $F$ . Also let $P(x, y)$ be a point in the plane and $PM$ be the perpendicular distance of $P$ from $L$ .
The set of all points $P(x, y)$ such that

$\frac{\left|PM\right|}{\left|PF\right|}=e>1$

is called a hyperbola.
$F$ and $L$ are respectively focus and directrix of the hyperbola $e$ is the eccentricity.

Standard Equation of Hyperbola

Let $F(c, 0)$ be the focus with $c > 0$ and $x=\frac c{e^2}$ be the directrix of the hyperbola.
Also, let $P(x, y)$ be a point on the hyperbola, then by definition

$\frac{\left|PM\right|}{\left|PF\right|}=e$ .

$⇒\;\;\;\left|PM\right|=e\left|PF\right|$ .

$⇒\;\;\;\sqrt{(x-c)^2+(y-0)^2}=e\;(x-\frac c{e^2})$ .

$⇒\;\;\;(x-c)^2+(y-0)^2=e^2\;(x-\frac c{e^2})^2$ .

$⇒\;\;\;x^2-2cx+c^2+y^2=e^2x^2-2cx+\frac{c^2}{e^2}$ .

$⇒\;\;\;x^2+c^2+y^2=e^2x^2+\frac{c^2}{e^2}$ .

$⇒\;\;\;e^2x^2-x^2-y^2=c^2-\frac{c^2}{e^2}$ .

$⇒\;\;\;x^2(e^2-1)-y^2=c^2(1-\frac1{e^2})$ .

$⇒\;\;\;x^2(e^2-1)-y^2=\frac c{e^2}^2(e^2-1)\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; (2)$ .

let us set $a=\frac ce$ , so that $(2)$ become

$⇒\;\;\;x^2(e^2-1)-y^2=a^2(e^2-1)$ .

$⇒\;\;\;x^2(e^2-1)-y^2-a^2(e^2-1)=0$ .

$⇒\;\;\;\frac{x^2}{a^2}-\frac{y^2}{a^2(e^2-1)}-1=0$ .

$⇒\;\;\;\frac{x^2}{a^2}-\frac{y^2}{a^2(e^2-1)}=1$ .

$⇒\;\;\;\boxed{\frac{x^2}{a^2}-\frac{y^2}{b^2}=1}\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;(3)$ .

where $b^2=a^2(e^2-1)=c^2-a^{2\;}\;\;\;\;\;\;\;\;\;\;\;\;\;\because c=ae$ .

$Eq(3)$ is standard equation of Hyperbola

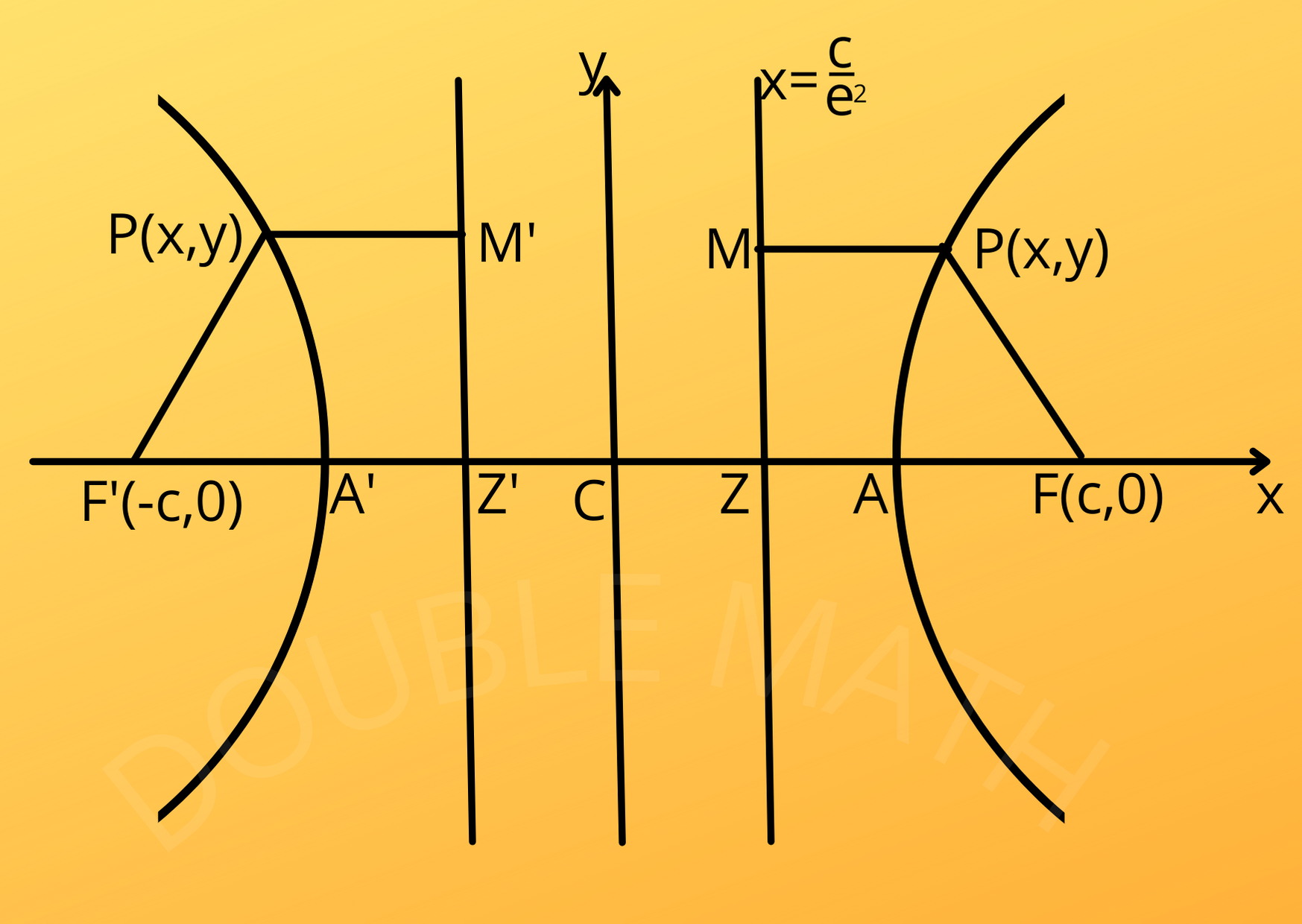
It is clear that the curve is symmetric with respect to both axes. If we take the point $(-c, 0)$ as focus and the line $x=\frac c{e^2}$ as directrix, then it is easy to see that the set of all points $P(x, y)$ such that

$\left|PF\right|=e\;\left|PM\right|$
is a hyperbola with $(3)$ as its equation.
Thus a hyperbola has two foci and two directrices.
If the foci lie on the $y-axis$ , then roles of $x$ and $y$ are interchanged in (3) and the equation
of the hyperbola becomes

$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ .

Definition: The hyperbola $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1...........................(1)$ .

meets the x-axis at points with $y = 0$ and $x = ±a$ . The points $A(-a, 0)$ and $A'(a, 0)$ are called vertices of the hyperbola. The line segment $AA' = 2a$ is called the transverse (or focal) axis of the hyperbola $(3)$ . The equation $(3)$ does not meet the y-axis in real points. However the line segment joining the points B(0, -b) and B’(0, b) is called the conjugate axis of the hyperbola. The midpoint $(0,0)$ of $AA'$ is called the centre of the hyperbola.
In the case of hyperbole (3), we have

$b^2=a^2(e^2-1)$ . The eccentricity $e=\frac ca>1$

so that, unlike the ellipse, we may have $b > a$ or $b < a$ or $b = a$

The point $(a \;sec \theta, b\; tan \theta)$ lies on the hyperbola $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ for all real values of $\theta$ . The equations $x = a \;sec \theta, \;\;\;y = b \;tan \theta$ are called parametric equations of the hyperbola.

Since

$y=\pm\frac ba\sqrt{x^2-a^2}\;\;\;\;\;\;$ when $\;\;\;\;\;\; x^2-a^2=x^2$

$y=\pm\frac bax\;\;\;\;\;\;i.e,\;\frac{x^2}{a^2}-\frac{y^2}{b^2}=0………..(2)$

The lines (2) do not meet the curve but the distance of any point on the curve from any of the two lines approaches zero. Such lines are called asymptotes of a curve. The joint equation of the asymptotes of (3) is obtained by writing 0 instead of 1 on the right-hand side of the standard form (3). Asymptotes are very helpful in graphing a hyperbola. The ellipse and hyperbola are called central conics because each has a center of symmetry.

Leave a Comment Cancel Reply