Binomial Theorem:
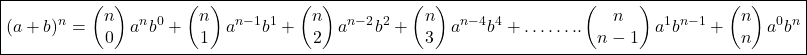
where ![]() and
and ![]() are real numbers and
are real numbers and ![]()
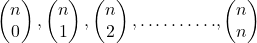 are binomial cofficient.
are binomial cofficient.
 and
and 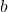 are exponents and
are exponents and 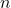 is called index.
is called index.
- The exponent of
 decreases from index to zero.
decreases from index to zero.
- The exponent of
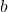 increases from zero to index.
increases from zero to index.
- The degree of each terms is equal to its index.
- The numbers of terms in the expansion is one greater than its index.
Example
Using Binomial Theorem:
![]()
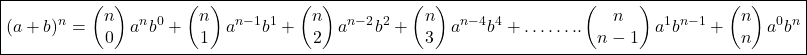
HERE
![]()
![]()
![]()

put in above expansion.
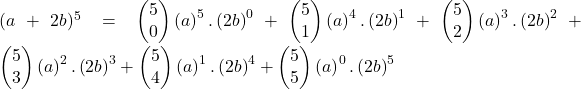
![]()
![]()
you can also see roles theorem
