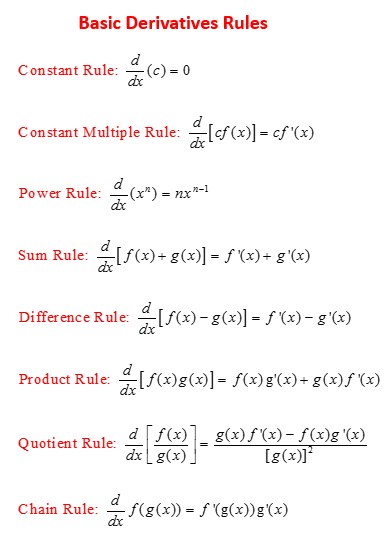
Sum and difference rule of derivative
Theorem:
Let ![]() and
and ![]() are differentiable at
are differentiable at ![]() ,
,
Then (![]() ) and (
) and (![]() ) are also differentiable at
) are also differentiable at ![]() and
and
![]()
That is
![]()
also
![]()
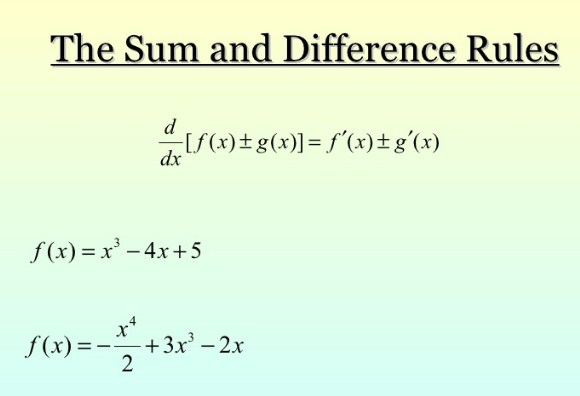
That is
![]()
Proof
You can prove sum and difference derivatives in easy way by using following pattren. Step 1: Let functionStep 2: adding
and
in
and
component respectively
Step 3: Subtracting
Step 4: Dividing
on both sides. Step 5: applying
on both sides.
Step 1:
Let ![]()
Step 2: Addingon both sides
![]()
Step 3: Subtracting 
![]()
![]()
![]()
![]()
Step 4: Dividing  on both sides
on both sides
![]()
Step 5: Taking  on both sides
on both sides
![]()
![]()
![]()
![]()
Example 1:
Sum and difference rule of derivatives
![]() and
and ![]()
Sum of derivatives
![]()
![]()
![]()
difference of derivatives
![]()
![]()
![]()
Example 2:
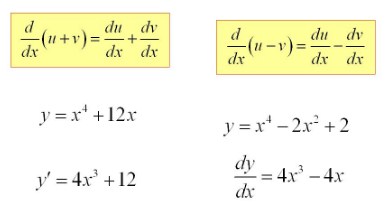
sum and difference rule of derivative
![]() and
and ![]()
Sum of derivatives
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
for computer information click here
