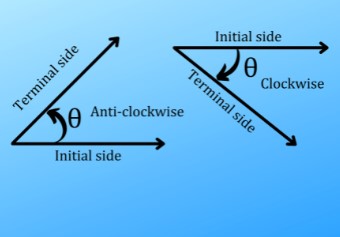
Angle in Math: Two rays with a common starting point form an angle. One of the rays of angle is called the initial side and the other is the terminal side. The angle is identified by showing the direction of rotation from the initial side to the terminal side.
An angle is said to be positive/negative if the rotation is anti-clockwise/clockwise. Angles are usually denoted by Greek letters such as ![]() (alpha),
(alpha), ![]() (beta),
(beta),![]() (gamma),
(gamma), ![]() (theta) etc.
(theta) etc.
There are two commonly used measurements for angle (in math): Degrees and Radians. which are explained as below:
- Sexagesimal System:(Degree, Minute and Second)
If the initial ray ![]() rotates in an anti-clockwise direction in such a way that it coincides with itself, the angle then formed is said to be of 360 degrees (
rotates in an anti-clockwise direction in such a way that it coincides with itself, the angle then formed is said to be of 360 degrees (![]() )
)
One rotation (anti-clockwise) = ![]()
![]() rotation (anti-clockwise) = 180° is called a straight angle
rotation (anti-clockwise) = 180° is called a straight angle![]() rotation (anti-clockwise) = 90° is called a right angle
rotation (anti-clockwise) = 90° is called a right angle
1 degree (1°) is divided into 60 minutes (60′) and 1 minute ( 1’) is divided into 60 seconds
(60′′). As this system of measurement of angle owes its origin to the English and because 90,
60 are multiples of 6 and 10, so it is known as the English system or the Sexagesimal system.
Thus
1 rotation (anti-clockwise) = 360°.
One degree (1°) = 60’
One minute (1′) = 60”
- Circular System (Radians)
There is another system of angular measurement, called the Circular System. It is most useful for the study of higher mathematics. Especially in Calculus, angles are measured in radians.
Definition: Radian is the measure of the angle subtended at the center of the circle by an arc, whose length is equal to the radius of the circle.
Consider a circle of radius r. Construct an angle ∠AOB at the center of the circle whose rays cut of an arc ![]() on the circle whose length is equal to the radius
on the circle whose length is equal to the radius ![]() .
.
Thus m AOB ∠ = 1 radian
